
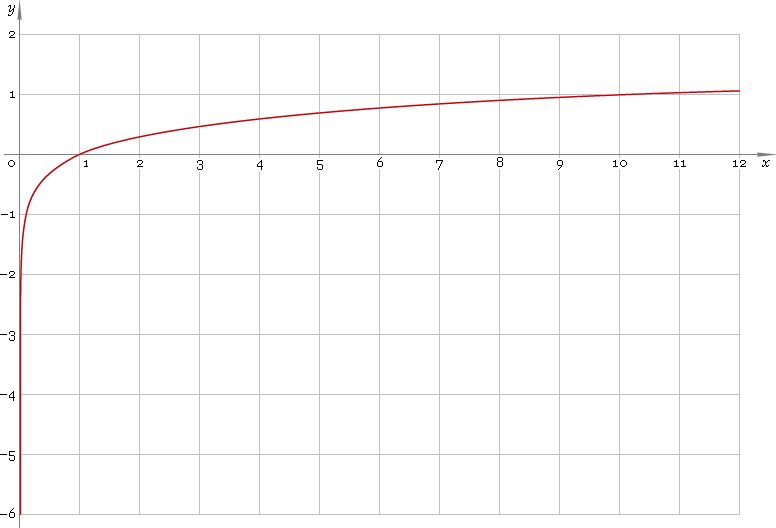
Then you must include on every digital page view the following attribution: If you are redistributing all or part of this book in a digital format, Then you must include on every physical page the following attribution: If you are redistributing all or part of this book in a print format, Want to cite, share, or modify this book? This book uses the When the graph approaches the y-axis so very closely but will never cross it, we call the line x = 0, x = 0, the y-axis, a vertical asymptote. We write the range in interval notation as ( − ∞, ∞ ). What is the range for each function? From the graphs we can see that the range is the set of all real numbers. We write the domain in interval notation as ( 0, ∞ ). What is the domain of the function? The graph never hits the y-axis. Now we will see that many characteristics of the logarithm function are simply ’mirror images’ of the characteristics of the corresponding exponential function. This makes sense as −1 = log a 1 a −1 = log a 1 a means a −1 = 1 a, a −1 = 1 a, which is true for any a. Notice too, the graph of each function y = log a x y = log a x also contains the point ( 1 a, −1 ). This makes sense as 1 = log a a 1 = log a a means a 1 = a. The graph of each function, also contains the point ( a, 1 ). This make sense because 0 = log a 1 0 = log a 1 means a 0 = 1 a 0 = 1 which is true for any a. We notice that for each function the graph contains the point ( 1, 0 ). The graphs of y = log 2 x, y = log 2 x, y = log 3 x, y = log 3 x, and y = log 5 x y = log 5 x are the shape we expect from a logarithmic function where a > 1. We use the notation f −1 ( x ) = log a x f −1 ( x ) = log a x and say the inverse function of the exponential function is the logarithmic function. To deal with this we define the logarithm function with base a to be the inverse of the exponential function f ( x ) = a x. f ( x ) = a x y = a x x = a y Solve for y. Oops! We have no way to solve for y ! Rewrite with y = f ( x ). If we try our algebraic method for finding an inverse, we run into a problem. This means an exponential function does have an inverse. Subtracting ‘undoes’ addition, multiplication ‘undoes’ division, taking the square root ‘undoes’ squaring.Īs we studied the exponential function, we saw that it is one-to-one as its graphs pass the horizontal line test. It works well to ‘undo’ an operation with another operation.
We have spent some time finding the inverse of many functions. If you missed this problem, review Example 2.2.


 0 kommentar(er)
0 kommentar(er)
